Formulas of the capacitance of parallel plate capacitors
Last updated on April 18th, 2022 at 05:32 pm
In this post, we will list down a few important formulas that we use to calculate the capacitance of the parallel plate capacitors. But before that, we will have a quick revision of the fundamentals.
A parallel-plate capacitor is a pair of two conductors of any shape which are close to each other and have equal and opposite charges.
A capacitor is an arrangement that can store a sufficient quantity of charge.
The quantity of charge that can be given to a parallel-plate capacitor is limited by the fact that every dielectric medium becomes conducting at a certain value of the electric field.
The capacitance of a parallel-plate capacitor is Directly proportional to the area of the plates (A).
The capacitance of a parallel-plate capacitor is Inversely proportional to the distance between plates (d).
The capacitance of a parallel-plate capacitor is Directly proportional to the dielectric constant of the medium filled between its plates (K).
[ Read: Factors affecting the capacitance of the parallel-plate capacitors ]
Capacitance formulas of Parallel plate capacitors
Here are some important Formulas that we can use to calculate the capacitance of parallel plate capacitors.
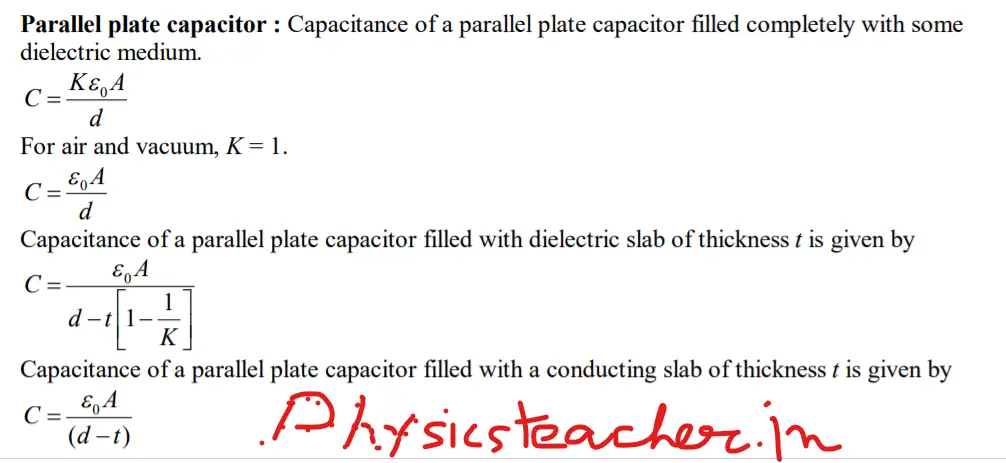
1 ] The capacitance of a parallel plate capacitor filled completely with some dielectric medium will have a capacitance C = K ε0 A /d.
2 ] For air and vacuum, K = 1. So, with air as the dielectric medium, the equation of the capacitance is
C = ε0 A /d
3 ] The capacitance of a parallel plate capacitor filled with a dielectric slab of thickness t is given by
C = ε0 A /[ d – t[1 – 1/k]]
4 ] The capacitance of a parallel plate capacitor filled with a conducting slab of thickness t is given by
C = ε0 A /(d – t)