Surface tension – numerical problems with solution
In this post, we will solve a few selected numerical problems from the Surface Tension chapter (Physics class 11).
Numerical problems from the Surface Tension chapter
1 ) A wire bent in the form of a ring of radius 5 cm rests on the surface of the water in a beaker.
A force of 4.4 gwt is required to pull the ring free from the surface of the water.
What is the surface tension of water? (g = 10 ms–2)
Solution:
A thin film of water is formed in contact with the ring. The force of S.T acts along the circumference of the ring.
The liquid touches ring along both inner and outer circumference.
The downward pull on ring due to S.T = 2 [2 π r S], where S is the surface tension of water. This is equal to 4.4 g force.
Therefore
4.4×10–3×10 = 2[2×3.14×5×10–2]S
or S = [ 4.4×10–3×10 ] /[ 2×2×3.14×5×10–2]
S = 0.070 Nm–1 = 70×10–3 Nm–1
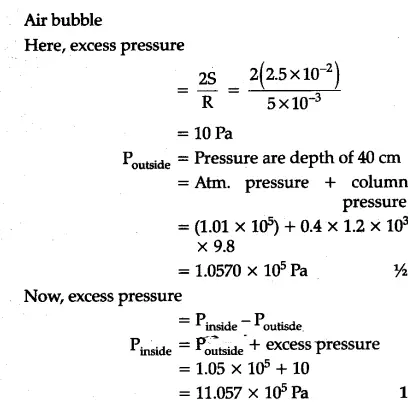
2) What is the excess pressure inside a bubble of soap solution of radius 5.00 mm, given that the surface tension of soap solution at the temperature of 20°C is 2.50 X 10-2 Nm-1 ?
If an air bubble of the same dimension were formed at a depth of 40.0 cm inside a container containing the soap solution (of relative density 1.20), what would be the pressure inside the bubble?
Solution: