Kirchhoff’s first law | Kirchhoff’s Current Law (KCL) – Explained & derived
Last updated on December 12th, 2021 at 09:40 am
Gustav Kirchhoff, an eminent German physicist is the person behind Kirchhoff’s first law dealing with electric current. This law is also known as Kirchhoff’s Current Law or KCL. The KCL states that, for any point or node in an electrical circuit, the sum of currents into that point is equal to the sum of currents out of that point.
[Know about Kirchoff’s Second Law (KVL)]
State Kirchhoff’s first law or KCL | Explain Kirchhoff’s first law with a diagram
Kirchhoff’s first law or current law (KCL) states that, for any point in an electrical circuit, the sum of currents into that point is equal to the sum of currents out of that point.
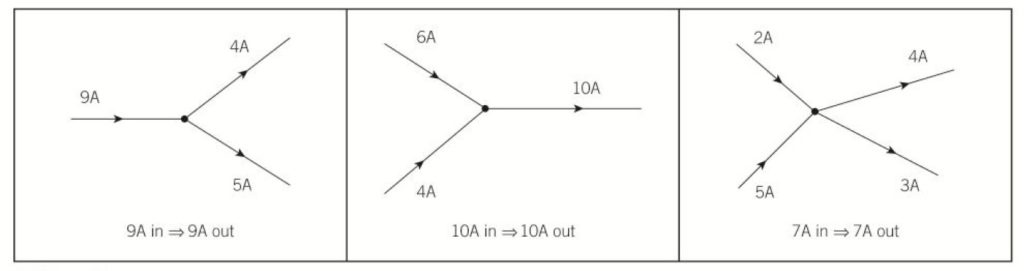
The diagram above will help to understand the first law of Kirchhoff. The law can be written as Σiin=ΣIout where Σ denotes ‘sum of’.
Σiin is the sum of the current into a point and ΣIout is the sum of current out of that point.
In diagram(1-a), 9 Ampere current is flowing into the node and then again (5+4) Ampere i.e. 9 Ampere current is coming out of the same node.
In diagram (1-b) total (6+4) Ampere = 10 Ampere current is coming into a node and again 10 Ampere current comes out from the node.
Lastly, in diagram (1-c) total current coming into the shown node is (5 +2 ) Amp = 7 Amp.
And total current coming out is also (4+3) Amp = 7 Amp. All these examples help to understand the first law of Kirchoff (KCL).
What is the Conservation of Charge Law?
Conservation of charge states that electric charge can neither be created nor destroyed. The total amount of electric charge in the universe is constant.
Deriving Kirchhoff’s first law from the Conservation of Charge Law
Kirchhoff’s first law can be derived from the Conservation of Charge Law. The charge is a fundamental physical property and one of the properties that must be conserved.
Kirchhoff’s first law is based on the Conservation of charge, where the charge (coulombs) is the product of the current (in amperes) and the time (in seconds). Charge can’t be destroyed, so the charge carriers entering a point in a given time must equal the total number of charge carriers leaving that same point during that time.
Mathematically, we can write the following equation to express the conservation of charge:
Total charge entering into a node = Total charge coming out of the node
=> Qentering = Qleaving ……………………..(1)
Now, as charge = current x time duration, we can write the following from equation (1)
=> Ientering. Δt= Ileaving. Δt
=> Ientering = Ileaving
This states that current coming into a point = current coming out of that point, which is exactly the expression of KCL. Thus we can derive Kirchhoff’s current law(KCL) or Kirchhoff’s first law taking help from the conservation of charge.
Parallel Circuit and Application of KCL
Let’s take a parallel circuit and find out how to use KCL for it.
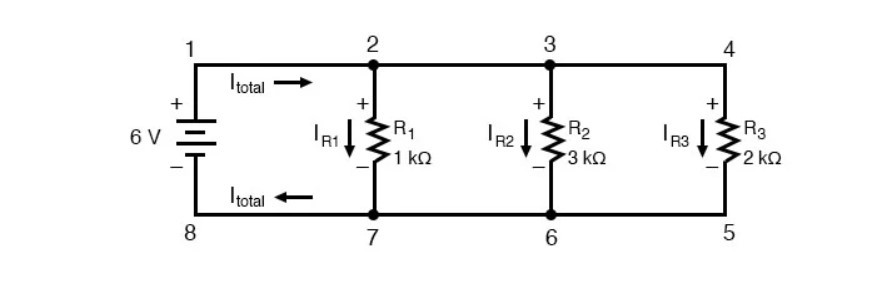
As per KCL, we can write the equations and solve them to find out individual current components flowing through different resistors.
