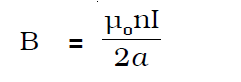Magnetic field along the axis of a circular coil carrying current

In this post, we will see how to derive equations for the Magnetic field along the axis of a circular coil carrying current. We will also find the magnetic field at the center of a circular coil.

Magnetic field along the axis of a circular coil carrying current – formula & its derivation

Let us consider a circular coil of radius ‘a’ with a current I as shown in the Figure below. P is a point along the axis of the coil at a distance x from the center O of the coil.

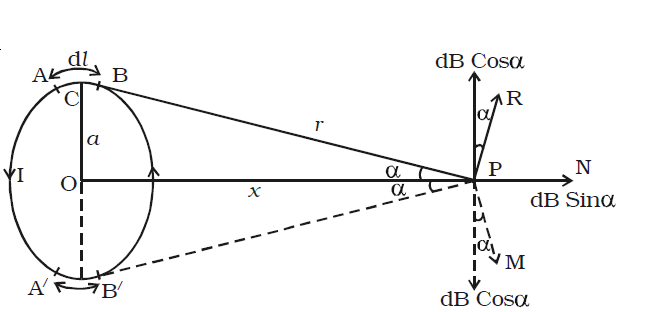
AB is an infinitesimally small element of length dl. C is the mid point of AB. And, CP = r

According to Biot-Savart law, the magnetic field at P due to the element dl is

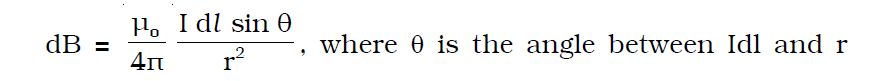
Here, θ = 90^o

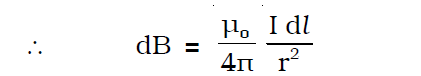
The direction of dB is perpendicular to the current element Idl and CP. It is therefore along PR perpendicular to CP.
Considering the diametrically opposite element A′B′, the magnitude of dB at P due to this element is the same as that for AB but its direction is along PM. Let the angle between the axis of the coil and the line joining the element (dl) and the point (P) be α.

dB is resolved into two components, dB sin α along OP and dB cos α perpendicular to OP.

dB cos α components due to two opposite elements cancel each other whereas dB sin α components get added up. So, the total magnetic field at P due to the entire coil is

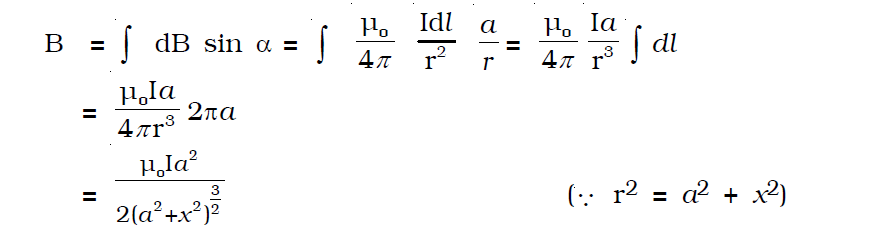
If the coil contains n turns, the magnetic field is

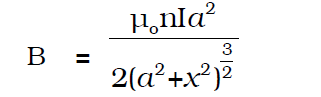
magnetic field at the center of a circular coil carrying current – formula & derivation

We have seen in the previous section that If the current-carrying circular coil contains n turns, the magnetic field is

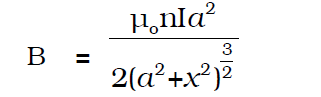
At the center of the coil where x = 0, the magnetic field B is