HOW TO SOLVE PROBLEMS in Physics? | How to solve numerical problems in physics? – A comprehensive Guide

Last updated on March 10th, 2022 at 02:48 pm

While guiding students with their physics syllabus often we face these queries from the students: “How to solve numerical problems in physics?” or “How to solve problems in physics?”. A similar question arises again while solving chemistry numerical as well. The activity of problem-solving in physics (or chemistry) is made easier by using certain techniques that help organize your thinking. One such technique is to follow a format, such as the following procedure we will discuss in detail in the next section of this post.

How to solve numerical problems in physics? – step-by-step procedure

If we want to solve problems in physics easily then we have to organize our thinking by using some techniques. One such technique is to follow a format, such as the following step-by-step procedure. So let’s discuss that in the next paragraphs.

Step 1: Read through the problem and make a list of the variables with their symbols on the left side of the page, including the unknown with a question mark.

Step 2: Inspect the list of variables and the unknown, and identify the equation that expresses a relationship between these variables. Write the equation on the right side of your paper, opposite the list of symbols and quantities.

Step 3: If necessary, solve the equation for the variable in question (using symbols only without data). That means this step must be done before substituting any numbers or units in the equation. This simplifies things and keeps down confusion that might otherwise result.
As an example, say you need to use the equation F = ma to find out the value of the mass, so in that case, the equation can be worked upon without putting the data first to take it to the form: m = F/a.

Step 4: If necessary, convert unlike units so they are all the same. For example, if a time is given in seconds and a speed is given in kilometers per hour, you should convert the km/h to m/s. Again, this step should be done at this point in the procedure to avoid confusion or incorrect operations in a later step.

Step 5: Now you are ready to substitute the number value and unit for each symbol in the equation (except the unknown). Note that it might sometimes be necessary to perform a “subroutine” to find a missing value and unit for a needed variable.

Step 6: Do the indicated mathematical operations on the numbers and on the units. This is easier to follow if you first separate the numbers and units, as shown in the example that follows. Then perform the indicated operations on the numbers and units as separate steps, showing all work.

Step 7: Now ask yourself if the number seems reasonable for the question that was asked, and ask yourself if the unit is correct. For example, 250 m/s is way too fast for a running student, and the unit for speed is not liters.

Step 8: Draw a box around your answer (numbers and units) to communicate that you have found what you were looking for. The box is a signal that you have finished your work on this problem.

Explaining the procedure – how to solve physics problems

1 ) Read the problem carefully, perhaps several times, to understand the problem situation. Make a sketch to help you visualize and understand the problem in terms of the real world.

2 ) Be alert for information that is not stated directly. For example, if a moving object “comes to a stop,” you know that the final velocity is zero, even though this was not stated outright. Likewise, questions about “how far?” are usually asking a question about distance, and questions about “how long?” are usually asking a question about time.

Such information can be very important in procedure step 1 listed in the section above, the listing of quantities and their symbols. Overlooked or missing quantities and symbols can make it difficult to identify the appropriate equation.

3 ) Understand the meaning and concepts that an equation represents. An equation represents a relationship that exists between variables. Understanding the relationship helps you to identify the appropriate equation or equations by inspection of the list of known and unknown quantities (procedure step 2 in the section above).

4 ) Solve the equation before substituting numbers and units for symbols (procedure step 3 in the section above).

5 ) Note whether the quantities are in the same units. A mathematical operation requires the units to be the same; for example, you cannot add nickels, dimes, and quarters until you first convert them all to the same unit of money.

Likewise, you cannot correctly solve a problem if a time quantity is in seconds and another time quantity is in hours. The quantities must be converted to the same units before anything else is done (procedure step 4 in the section above).

6 ) Perform the required mathematical operations on the numbers and the units as if they were two separate problems (procedure step 6). You will find that following this step will facilitate problem-solving activities because the units you obtain will tell you if you have worked the problem correctly. If you just write the units that you think should appear in the answer, you have missed this valuable self-check.

7 ) Be aware that not all learning takes place in a given time frame and that solutions to problems are not necessarily arrived at “by the clock.” If you have spent half an hour or so unsuccessfully trying to solve a particular problem, move on to another problem or do something entirely different for a while.

Problem-solving often requires time for something to happen in your brain. If you move on to some other activity, you might find that the answer to a problem that you have been stuck on will come to you “out of the blue” when you are not even thinking about the problem. This unexpected revelation of solutions is common to many real-world professions and activities that involve thinking.

Example Problem to solve

As we have gone through the step-by-step procedure to understand how to solve the problems in Physics, now it’s time to implement those steps we just learned while solving a sample numerical problem. The next section of this just does this. So let’s go ahead and solve a simple problem.

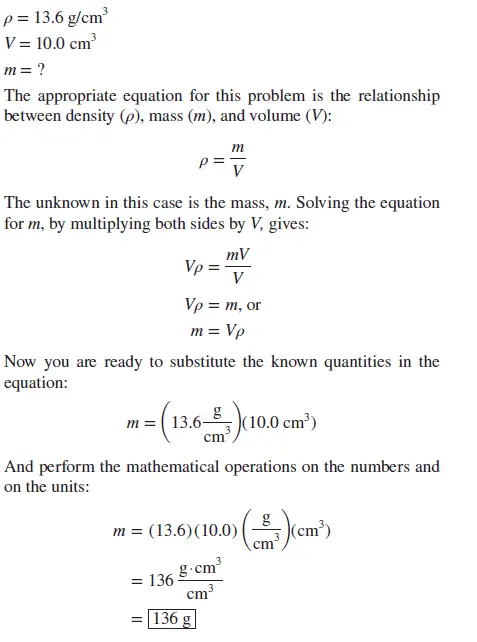
Physics problem statement: Mercury is a liquid metal with a mass density of 13.6 g/cm3. What is the mass of 10.0 cm^3 of mercury?

Solution
The problem gives two known quantities, the mass density (ρ) of mercury and a known volume (V), and identifies an unknown quantity, the mass (m) of that volume. Make a list of these quantities:

Summary

We have presented a step-by-step guide to help students understand how to solve problems in physics with confidence. Also here we have solved a sample problem to implement the procedure we learned.

Now we suggest you visit our page on numerical problems in physics and solve the problems listed under the posts (as per your syllabus or requirement). This is the link to that page that lists the numerical posts.