Instantaneous Acceleration – definition & formula with solved problem

Last updated on May 8th, 2021 at 09:54 am

The quantity that tells us the rate at which an object is changing its velocity at a specific instant in time anywhere along its path is the instantaneous acceleration.

That means when we say ‘acceleration’ of a body we actually mean instantaneous acceleration.

Another related term is the average acceleration that is basically for a duration of time and we have discussed in another post.

What do we mean by instantaneous acceleration?

Instantaneous acceleration is a quantity that tells us the rate at which an object is changing its velocity at a specific instant in time anywhere along its path. This is also called acceleration as well.

Definition of instantaneous acceleration

The instantaneous acceleration of an object is the limit of the average acceleration as the elapsed time approaches zero, or the derivative of velocity v with respect to t:
a(t) = dv(t)/dt.

Derive the Formula of instantaneous acceleration (step by step)

Instantaneous acceleration is the average acceleration between two points on the path in the limit that the time (and therefore the displacement) between the two points approaches zero.

We will use the general formula of average acceleration to find out the formula of Instantaneous acceleration with the tweak of making the time elapsed nearly zero.

To illustrate this idea mathematically, we need to express velocity v as a continuous function of t denoted by v(t).

The expression for the average acceleration between two points using this notation is
a = [v(t2) − v(t1)] / (t2 − t1)

To find the instantaneous acceleration at any position, let’s consider the following:
Say, t1 = t and t2 = t + Δt.

As said earlier above, this Δt has to be near zero if we want to calculate instantaneous acceleration.

After inserting these expressions into the equation for the average acceleration and taking the limit as Δt → 0, we find the expression for the instantaneous acceleration:

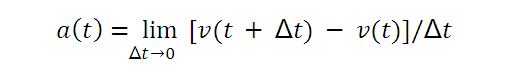
a(t) = dv(t)/dt …………(1)
This is the required equation or formula we are looking for.

So How to find acceleration while solving a numerical? | How to get instantaneous acceleration using formula?

By the term acceleration actually, we mean Instantaneous acceleration.
So by using the equation a(t) = dv(t)/dt, we can easily find the value of acceleration or instantaneous acceleration.

Sample numerical problems on instantaneous acceleration physics – solved

Q1.) The position of a particle is given by x(t) = 3.0t + 0.5t³ m .
a. find the instantaneous acceleration at t = 2.0 s.

Solution:
Here, x(t) = 3.0t + 0.5t³ m

So, v(t) = dx(t)/dt = 3.0 + 1.5t² m/s .

Therefore, a(t) = dv(t)/dt = 3 t m/s^2………..(a)

So at t = 2 seconds, instantaneous acceleration is 3t = 3.2 m/s^2 = 6 m/s^2

---

Also Read: (suggested reading)

Difference between Instantaneous Speed and Instantaneous Velocity

Difference between average speed and average velocity

Instantaneous Velocity – definition & equation with solved problem

Average velocity – definition, formula

Instantaneous Acceleration – definition & formula with solved problem

Average Acceleration and its formula & solved numerical problems