Force and Laws of Motion Class 9 Numericals
Last updated on July 5th, 2023 at 03:15 pm
This page presents a set of Force and Laws of Motion class 9 Numericals. We have used linear motion equations and force equations to solve these numerical problems.
[detailed solution of the numerical questions is given]
Force and Laws of Motion Class 9 Numericals – solved numerical problems
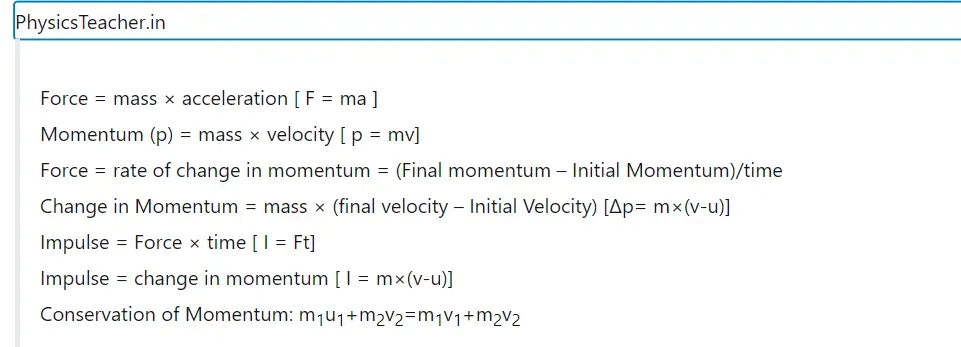
In the next section, we will find a list of formulas that we will use to solve the numerical problems in this post. Then we will solve the sets of physics numerical problems from class 9 Force and Laws of Motion chapter.
Formulas Used
PhysicsTeacher.in
Force = mass × acceleration [ F = ma ]
Momentum (p) = mass × velocity [ p = mv]
Force = rate of change in momentum = (Final momentum – Initial Momentum)/time
Change in Momentum = mass × (final velocity – Initial Velocity) [Δp= m×(v-u)]
Impulse = Force × time [ I = Ft]
Impulse = change in momentum [ I = m×(v-u)]
Conservation of Momentum: m1u1+m2v2=m1v1+m2v2
Force and Laws of Motion Numericals class 9 – question and solution
1> A force of 10 N is applied for 0.1 seconds on a body of mass 1 kg initially at rest. The force then ceases to act. What would be the velocity of the body just after this? After 2 more seconds with what velocity the body will move?
A force will cause an acceleration a.
a = force/mass = 10/1 m/s^2 = 10 m/s^2
The force acts for 0.1 seconds. That means the body will remain under an accelerated motion for 0.1 sec.
The velocity acquired post this interval of 0.1 s is V
And, V =u + at = 0 + 10. 0.1 = 1 m/s
As there is no force after this interval, the body will continue its motion with a uniform velocity of 1 m/s.
So after 2 more seconds, its velocity is 1 m/s.
2> A force acts for 2 seconds on a body of mass 2 kg initially at rest. Just after the force stops to act the body moves 10 m in the next 2 seconds. Find the magnitude of the force.
After the force stops to act, the velocity of the body is = V = distance/time=10/2 m/s = 5 m/s
Initial velocity = U =0
The time interval for the accelerated motion in presence of the force =t= 2 seconds
Therefore we can find the acceleration a
a= (V-U)/t =(5-0)/2 = 2.5 m/s^2
m=Mass of the body is 2 kg.
a=2.5 m/s^2
So force F = ma = 2 x 2.5 N= 5 N
3> Calculate the magnitude of force which when applied on a body of mass 0.5 kg produces an acceleration of 5 m/s2
Sol: Force = mass x acceleration = 0.5 x 5 N = 2.5 N
4> A ball of mass 0.01 kg is moving with a velocity of 50 m/s. On applying a constant force for 2 seconds on the ball, it acquires a velocity of 70 m/s.
Calculate the initial and final momentum of the ball.
Also, calculate the rate of change of momentum and the acceleration of the ball.
What would be the magnitude of the force applied?
Initial velocity = U = 50 m/s
Final velocity = V = 70 m/s
Time interval = 2 s
mass = m = 0.01 kg
Initial momentum = mU = 0.01 x 50 =0.5 kg m/s
Final momentum = mV = 0.01 x 70 = 0.7 kg m/s
Acceleration =a= (V-U)/t =(70-50)/2 m/s^2 = 10 m/s^2
Force = m a = 0.01 x 10 N = 0.1 N
5> An object of mass 1 kg is moving at a speed of 50 m/s. The object is brought to rest in 0.05 seconds by an external system. Find the change in momentum of the object and the average force applied by the external system.
Initial momentum = mU = 1 x 50 = 50 kg m/s
Final momentum = mV = 0 (as V =0)
So the change in momentum =mV – mU = 0 – 50 = – 50 kg m/s
Force = change of momentum / time = -50/0.05 N = – 1000 N
The negative sign denotes a force that opposes the motion, causing retardation.
6> A car of mass 500 kg moving at a speed of 36 Km/hr is stopped by applying brakes in 10 s. Calculate the force applied by the brakes.
Mass = 500 kg
initial velocity U = 36 kmph= 36 x (5/18) m/s =10 m/s
final velocity =0
time interval =10 s
Therefore, acceleration (retardation here actually) = (0-10)/10 m/s^2 = -1 m/s^2
The force applied by the brakes = mass x acceleration = 500 x (-1) N = -500 N
The negative sign of the force denotes that the force is resistive i.e. the force opposes the motion.
7> A bullet of mass 50 g moving with an initial velocity of 100 m/s, strikes a wooden block and comes to rest after penetrating a distance of 2 cm in it. Calculate
– the initial momentum of the bullet
-final momentum of the bullet
-retardation caused by the wooden block and
-resistive force applied by the wooden block
Initial momentum= mass x initial velocity
= (50/1000)kg x (100) m/s = 5 kg m/s
final momentum = 0 (as final velocity is zero)
To find Retardation a, we will use the following equation:
V^2 = U^2 – 2as
Putting values,
0=(100)^2 – 2a (2/100)
Retardation a= (10000x 100)/4 = 25 x 10^4 m/s^2
Resistive force = m a = (50/1000) x (25 x 10^4)=12500 N
8> A force when applied on a block of 10 kg mass produces an acceleration of 5 m/s^2.
When the same force is applied on a different block initially at rest then the block gains a velocity of 2 m/s in 1 second. What is the magnitude of the force in Newton and what is the mass of the second block?
1st case: F = ma = 10 x 5 N = 50 N
2nd case
F = same as first case = 50 N
U = 0
V = 2 m/s
t = 1 sec
So, Acceleration a =(V-U)/t = 2 m/s^2
Force = mass X acceleration
Mass of the second block = M = F/a = 50/2 Kg = 25 kg
9> Velocity of an object changes from 2 m/s to 10 m/s in 4 seconds.
If the mass of the object is 10 kg then how much force will be required to do this?
Acceleration a= (10-2)/4 = 2 m/s^2
Mass m = 10 kg
Hence, Force F = ma = 10 x 2 N = 20 N
10> A car is moving with a uniform velocity of 30 m/s. it is stopped in 2 seconds by applying a force of 1500 N through its brakes.
Calculate (a) the change in momentum of the car (b) the retardation produced in the car and (c) the mass of the car.
a) Change in momentum = F t = 1500 x 2 kg m/s = 3000 kg m/s
b) Acceleration a = (0-30)/2 = -15 m/s^2
This means, retardation = 15 m/s^2
c) Mass of the car m = force/magnitude of acceleration = F/a = 1500/15 = 100 kg
Force and Laws of Motion Numerical – practice problems
11> A boy pushes a wall with a force of 20 N toward the North. What force is exerted by the wall on the boy?
12> A block of mass 1.5 kg is hanging from rigid support by a string.
What is the force exerted by the
(i) block on the string
(ii) string on the block
(g = 10 m/s^2)
13> A stone of size 1 kg is thrown with a velocity of 20 m/s across the frozen surface of a lake and it comes to rest after traveling a distance of 50 m. What is the force of friction between the stone and the ice?
14> A body of mass 0.5 kg is resting on a frictionless surface.
When a force of 2000 dyne acts on it for 10 s, then calculate the distance traveled by it in 10 seconds.
15> A force of 10 N produces an acceleration of 5 m/s^ in mass m1 and 20 m/s^2 in mass m2. What will be the acceleration by this force, if both masses m1 and m2 are tied together?
Very Short Answer Type Questions (Numerical)
16) What is the force which produces an acceleration of 1 m/s2 in a body of mass 1 kg?
17) Find the acceleration produced by a force of 5 N acting on a mass of 10 kg.
18) A girl weighing 25 kg stands on the floor. She exerts a downward force of 250 N on the floor. What force does the floor exert on her?
19) If the mass of a body and the force acting on it are both doubled, what happens to the acceleration?
20) The mass of object A is 6 kg whereas that of another object B is 34 kg. Which of the two objects, A or B, has more inertia?
Short Answer Type Questions (Numerical)
21) What is the change in momentum of a car weighing 1500 kg when its speed increases from 36 km/h to 72 km/h uniformly?
22) Calculate the momentum of a toy car of mass 200 g moving with a speed of 5 m/s.
23) A body of mass 25 kg has a momentum of 125 kg.m/s. Calculate the velocity of the body.
24) A 60 g bullet fired from a 5 kg gun leaves with a speed of 500 m/s. Find the speed (velocity) with which the gun recoils (jerks backward).
25) A 10 g bullet traveling at 200 m/s strikes and remains embedded in a 2 kg target which is originally at rest but free to move. At what speed does the target move off?
26) A body of mass 2 kg is at rest. What should be the magnitude of force which will make the body move with a speed of 30 m/s at the end of 1 s?
27) A body of mass 5 kg is moving with a velocity of 10 m/s. A force is applied to it so that in 25 seconds, it
attains a velocity of 35 m/s. Calculate the value of the force applied.
28) A car of mass 2400 kg moving with a velocity of 20 m s–1 is stopped in 10 seconds by applying brakes.
Calculate the retardation and the retarding force.
29) How long will it take a force of 10 N to stop a mass of 2.5 kg which is moving at 20 m/s?
30) For how long should a force of 100 N act on a body of 20 kg so that it acquires a velocity of 100 m/s?
Long Answer Type Questions (numerical)
31) A 1000 kg vehicle moving with a speed of 20 m/s is brought to rest in a distance of 50 meters:
(i) Find the acceleration.
(ii) Calculate the unbalanced force acting on the vehicle.
32) A 150 g ball, traveling at 30 m/s, strikes the palm of a player’s hand and is stopped in 0.05 seconds. Find the force exerted by the ball on the hand.
33) An unloaded truck weighing 2000 kg has a maximum acceleration of 0.5 m/s2. What is the maximum
acceleration when it is carrying a load of 2000 kg?
Summary
We have completely solved a set of numerical problems from the Force and Laws of Motion chapter of the class 9 syllabus. We have presented here a bundle of practice problems from this chapter as well. We will also add solutions to those as well gradually. Hope you have found this post on Force and laws of motion class 9 Numericals useful enough. So please share this and come back soon for more numerical solutions.
 Motion – MCQ Question Set – you must try
Motion – MCQ Question Set – you must try
