Dielectric & Dielectric constant (Relative Permittivity)

Last updated on May 17th, 2022 at 01:58 pm

In this post, we will talk about the Dielectric & Dielectric constant (Relative Permittivity). One way to keep the plates of a capacitor apart is to insert an insulator called a dielectric between them. A dielectric increases the capacitance.

The action of a dielectric in a charged capacitor

A dielectric material is one that is non-conducting. If such a material is placed in an electric field the charges within its molecules separate, although this separation does not cause conduction (Figure 1).

If a dielectric material is placed between the plates of a charged capacitor, opposite charges will be induced on the two surfaces of the dielectric. This has the effect of reducing the electric field and the potential difference across the capacitor. As a result, the capacitance of the capacitor is increased. (Capacitance is inversely proportional with the potential difference). For an isolated capacitor, even after inserting the dielectric the stored charge remains same. But, the stored energy in the capacitor falls after introducing a dielectric.

If K = Dielectric constant, then

• New electric field after dielectric introduction: E_new=E_old/K
• New Potential Difference after dielectric introduction: V_new=V_old/K
• New Capacitance after dielectric introduction: C_new=KC_old
• For isolated capacitor: Stored Charge after dielectric introduction = Stored Charge before dielectric introduction
• Energy stored after dielectric introduction = Energy stored before dielectric introduction/K

How a dielectic reduces the electric field?

There is an electric field between the plates of a capacitor. This field polarizes the molecules in the dielectric; that is, some of the electrons in the molecules move to the end of the molecule, near the positive plate:

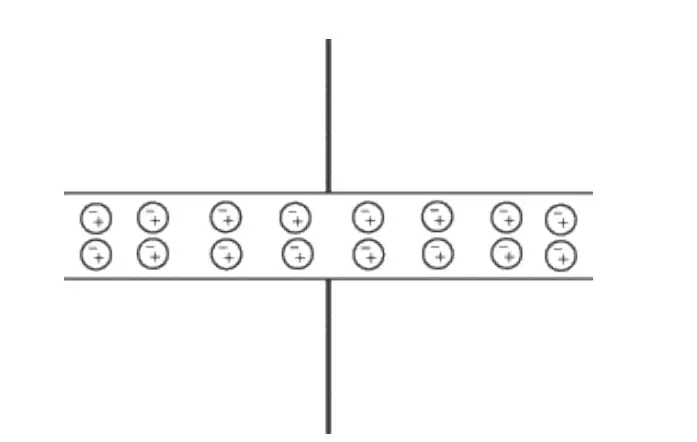
The movement of electrons creates a layer of negative charge by the positive plate and a layer of positive charge by the negative plate. This separation of charge, in turn, creates an electric field in the dielectric that is in the opposite direction of the original field of the capacitor. This reduces the total electric field:
E_new = E_old – E_induced = E_old/K

This K is called the dielectric constant, and it varies from material to material. The value of K for air and vacuum is 1. For other materials, K >1.
This dielectric constant (K) is also known as Relative permittivity(ε_r). [we will read about this in a dedicated section in this post]

How a dielectric increases the capacitance of a capacitor?

Introduction of a dielectric in a parallel plate capacitor, reduces the total electric field (E).

For a parallel-plate capacitor, the reduction in E means that V is also reduced by a factor of K.

V_new = V_old/K ……………. (1)

For an isolated capacitor Q remains unchanged.

This means, Charge stored in air capacitor = charge stored in capacitor with dielectric inserted, completely filling the separation between the plates

Initially, Q = C_old V_old …….(i) (for air-capacitor)

Later, when dielectric replaces air completely, Q = C_newV_new

=> Q = C_new.V_old/K …….. (ii)

From (i) and (ii), C_old V_old = C_new.V_old/K

=>C_new= K C_old

So, we find that C_new= K C_old. ………………….. (2)

As the value of K >1, C_new> C_old. Thus a dielectric increases the capacitance.

=> K = C_new / C_old ……………(3)

Hence, the dielectric constant K can also be expressed as:
K =Capacitance with dielectric between the plates / Capacitance with vacuum between the plates

For metals, K = ∞, for mica K ≈ 6, and for paper K = 3.6.

Relative permittivity | dielectric constant

It is often useful to compare the electrostatic properties of materials by using a quantity known as the relative permittivity (ε_r) – also known as the dielectric constant(K).

This relative permittivity or dielectric constant is defined using the relation:
permittivity (ε) = relative permittivity (ε_r) × permittivity of free space (ε₀)

The capacitance of a parallel-plate capacitor with a material of relative permittivity ε_r filling the space between the plates is:
capacitance (C) = ε_rε₀A/d

The relative permittivity of most gases is very nearly equal to 1.00, but for solids and liquids, it varies between 80 (water) and 2 (paraffin).

The relative permittivity (ε_r) can also be defined as the ratio of the capacitance when the space between the plates is a dielectric to that when there is a vacuum between the plates.