Voltage – Work done in separating charges

In this post, we discuss the concept of voltage (or Potential Difference) in terms of work done in separating charges.

Moving charges give rise to electricity. For charges to move, there must be some force to be applied on these charges, and this force can be caused by other positive or negative charges. Consider the situation shown in Fig. 1.

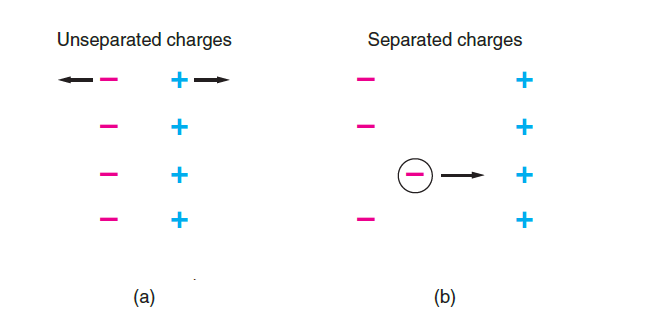
Let’s start out with some unseparated charges and then begin to separate them. If we try to pull the first negative charge to the left and the first positive charge to the right, It takes little work. But as try to move the next negative charge to the left, it is repelled by the negative charge already there, so more work is needed. Similarly, when we try to move the second positive charge to the right, it takes more work. And as more and more charges are separated, it takes more and more work.

Because work is done in separating the charges, there is electric potential energy. If a separated charge were free to move, then the electric potential energy is converted to the kinetic energy, and the said charge would move toward the charges of opposite sign. For example, a negative charge, as shown in Fig. 1 b, would move toward the positive charges. Electric potential energy would be converted into kinetic energy as required by the conservation of energy.

A related but different quantity called potential difference, or voltage, is most often considered Instead of electric potential energy, in this case. Voltage is defined as the amount of work it would take to move a charge between two points divided by the value of the charge. In other words, voltage (V) is the work (W) per unit charge (q), or the electric potential energy per unit charge.

Voltage = Work/charge

or, V = W/q

Note the symbol for voltage (caused by a separation of charge) is an italic “vee” (V), whereas the symbol for the volt unit is a roman “vee” (V).