The Transformer equation & Transformer efficiency
In this post we will discuss in detail about the transformer equation and transformer efficiency. We will also solve a few numerical problems using the transformer and efficiency equations.
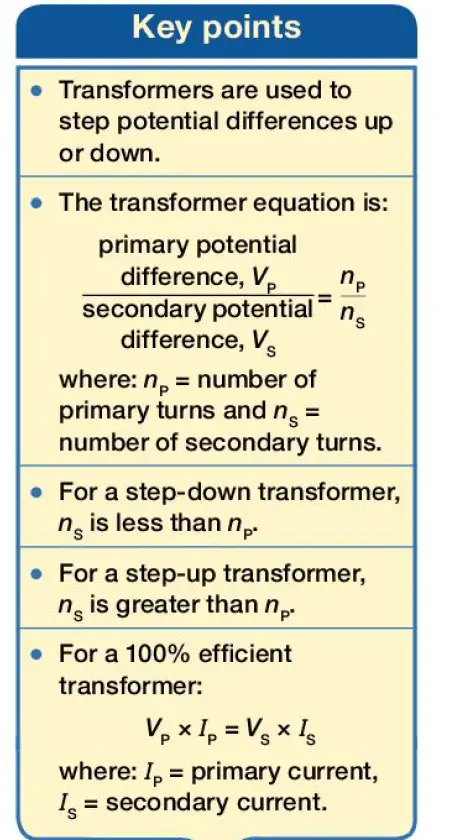
The Transformer equation
The secondary potential difference of a transformer depends on the primary potential difference and the number of turns on each coil. You can use the following equation to calculate any one of these factors if you know the other factors:
(Potential difference across primary, Vp) / (Potential difference across secondary, Vs) = (number of turns on primary, Np) / (number of turns on secondary, Ns)
=> Vp/Vs=Np/Ns
This equation is called the Transformer Equation]
For a step-up transformer, the number of secondary turns, Ns, is greater than the number of primary turns, Np. Therefore, Vs > Vp.
For a step-down transformer, the number of secondary turns, Ns, is less than the number of primary turns, Np. Therefore, Vs < Vp.
Worked example (numerical) using the Transformer equation
A transformer is used to step a potential difference of 230 V down to 10 V. The secondary coil has 60 turns. Calculate the number of turns of the primary coil.
Solution: Vp = 230 V. Vs =10V. Ns=60. Np = ?
Using, Vp/Vs=Np/Ns
=> Np = (Vp/Vs). Ns = (230/10). 60 = 1380 turns.
Transformer efficiency
Transformers are almost 100% efficient. When a device is connected to the secondary coil (see figure ), almost all the electrical power supplied to the transformer is delivered to the device. Now if we know how much electrical power a device connected to the secondary needs to work normally (i.e., the transformer output power), then this tells us how much electrical power needs to be supplied to the transformer (i.e., the transformer input power).

Power supplied to transformer = Input power = Primary Potential difference (Vp) x Primary current (Ip)
Power delivered by transformer = output power = secondary Potential Difference (Vs) x secondary current (Is)
If we can assume 100% efficiency (i.e., power supplied = power delivered), then:
Power supplied to transformer = Power delivered by transformer
Vp x Ip = Vs x Is
Worked Example (numerical) using transformer efficiency
Q: A step-down transformer is used to step down an alternating potential difference of 230 V to 12 V to supply electricity to a 12 V, 2.5 A lamp. Calculate the current in the primary coil of the transformer when the device is switched on. Assume that the transformer is 100% efficient.
Solution: Vp = 230 V, Vs = 12 V, Is = 2.5 A. Ip = ?
Vp Ip = Vs Is
=> Ip = Vs Is / Vp = 12 x 2.5/230 = 0.13 A
Take Away
In this post, we have discussed the Transformer equation and how it works with step-up and step-down transformers. We also discussed the transformer efficiency and derived an equation using the concept that Power supplied to transformer = Power delivered by transformer. We also solved numerical problems using equations related to transformer. Also you are suggested to read this post on how a transformer works & step up and step down concepts in power grids.
